
How to Use the Segment Addition Postulate to Show that ae=ab+bc+cd+de? For example, if AB = 3, BC = x, and AC = 5, then we can find x by subtracting AB from AC. If we have a missing length, let's say x, and we know the total length and the length of the other part of the segment, then we can apply the segment addition postulate to find x. How to Solve for x with Segment Addition Postulate? The segment addition postulate has its applications in construction, architecture, designing, etc. It can be used to find the sum of the smaller parts of a segment to find the total length. We can apply this postulate in calculating the missing lengths. What is a Segment Addition Postulate Used For? This is one of the examples of segment addition postulate. If the length of one part is 14 inches, it means the length of the other part of the rod is 30 - 14 = 16 inches. What are the Examples of Segment Addition Postulate?Īs per the segment addition postulate, if we have an iron rod of length 30 inches, and it is cut into two parts.
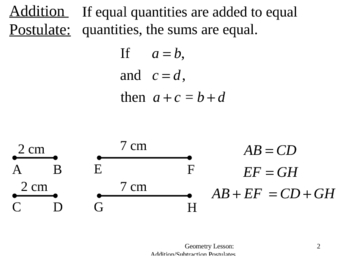
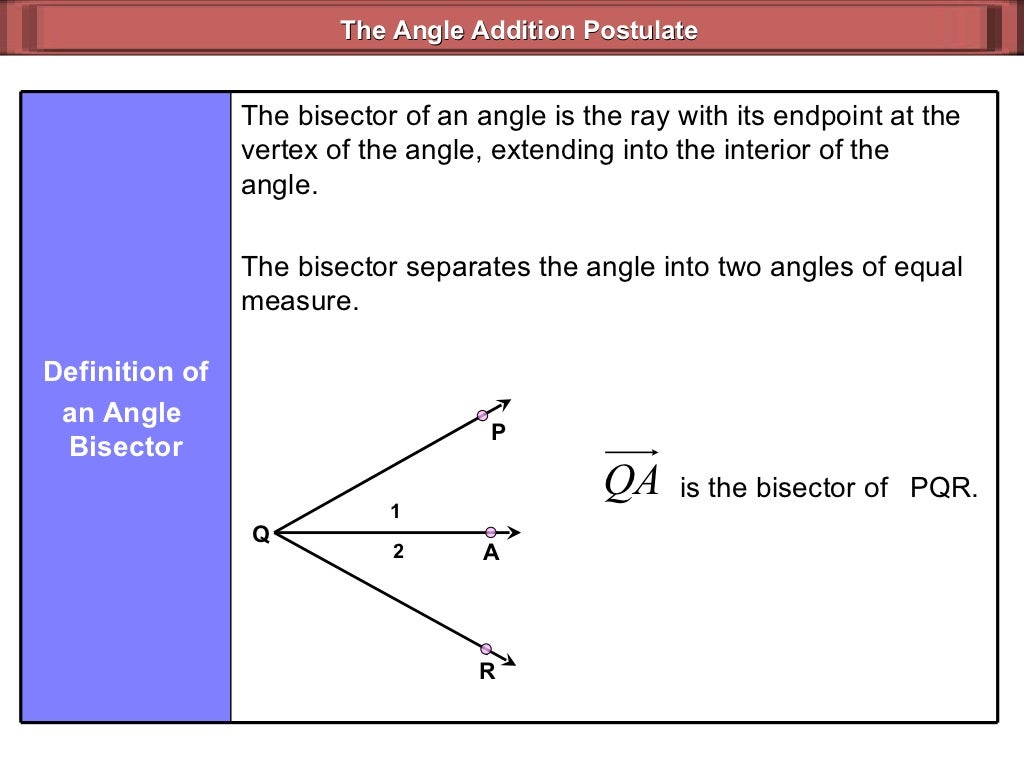
The distance between MP and PN must be equal to MN.A point P lies on a segment MN if and only if points M, P, and N are collinear.The two conditions of the segment addition postulate are given below: What are the Two Conditions of the Segment Addition Postulate? It is a mathematical fact that can be accepted without proof. So, if we have three collinear points A, B, and C on segment AC, it means AB + BC = AC. The segment addition postulate in geometry is the axiom which states that a line segment divided into smaller pieces is the sum of the lengths of all those smaller segments. FAQs on Segment Addition Postulate What is Segment Addition Postulate in Geometry?


 0 kommentar(er)
0 kommentar(er)
